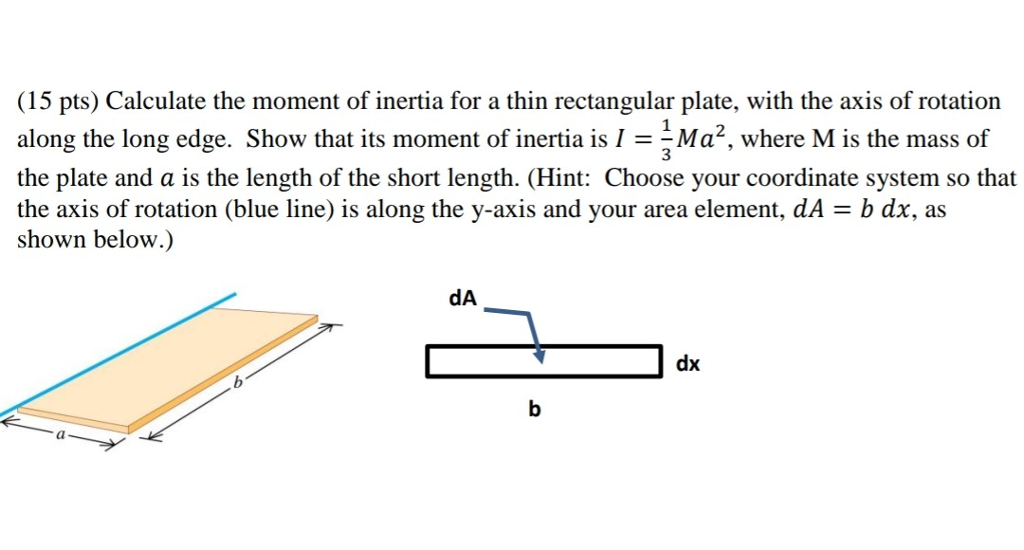
Rectangular Plane Mass Moment of Inertia Calculator. 15 pts Calculate the moment of inertia for a thin rectangular plate with the axis of rotation along the long edge.
Solved 15 Pts Calculate The Moment Of Inertia For A Thin Chegg Com
For an axis of rotation perpendicular to the plate through its center b.

. An Axis Passing Through Its Base. The Moment of Inertia for a Thin Rectangular Plate calculator compute the moment of inertia based on the mass and two dimensions of the plate height and width. A thin rectangular plate rotating on an axis along one edge of the plate with mass M and side lengths a and b where a is the distance perpendicular to the axis of rotation has a moment of inertia determined by the formula.
Viewed from Beside the Axis. The calculator return the moment of inertia in kilogram. Moment of inertia of the thin-walled cylinder about central axis with a radius 1 m and mass 2 kg.
Thus its moment of inertia will also be ma212 mass is distributed at a distance a2 from the axis where m is the mass of the plate and a is its side length. Then the distance from xy to the axis of rotation passing through 00perpendicular to the plate is x 2 y 2 and the moment of inertia is itexint_-a2a2int_-b2b2rho x2 y2dydx itex. I e m 12h2 w2 I e m 12 h 2 w 2 where.
I112 Ma²b² Thin rectangular plate axis along edge. Slender rod axis through one end. In this problem take the center of the rectangle to be at 00 so the vertices are a2 b2 etc.
Mass of the elemental section. M 945 g. Moment of inertia of the thin uniform rod through center with a length 3 m and mass 4 kg.
M m mass. M ρ x bdt. 11 rows Therefore the moment of inertia of a rectangular plate about a line parallel to an edge and.
Choose your coordinate system so that the axis of rotation blue line is along the y-axis and your area element 𝑑𝐴 𝑏 𝑑𝑥 as shown below. Rectangular Plane Mass Moment of Inertia Calculator. Rectangular Plates Thin Rods and Boards Description.
Rectangular Plate Mass Moment of Inertia Calculator About Edge Design Variables Length L in mm m lbs g Results I lbm-in 2 g-mm 2. The Moment of Inertia for a thin rectangular plate with the axis of rotation at the end of the plate is found using the following formula. Rectangular plate axis through center.
Length of the rectangle a 9 cm. I ρ b dy ty 2. A length both sides in mm m mass of sphere lbm kg Rectangular Plate Mass Moment of Inertia Calculator on Edge.
The mass of the rectangular plate is calculated as. Now we will find the mass moment of inertia of the elemental strip about the x-axis. Choose units and enter the following.
M 15 gcm² x 63 cm². Show that its moment of inertia is 𝐼 1 3 𝑀𝑎 2 where M is the mass of the plate and 𝑎 is the length of the short length. DM ρ x btdy.
The moment of inertia of thin uniform rectangular plate relative to the axis passing perpendicular to the plane of the plate through one of its vertices if the sides of the plate are equal to a and b and mass m is I m x a 2 b 2. I bh 3 12. Similarly the moment of inertia of the plate about the axis passing through the center and parallel to y-axis will also be ma212.
H h height. A 9 cm x 7 cm 63 cm². Width of the rectangle b 7 cm.
Moment of inertia of the thin ring about diameter with a radius 1 m and mass 2 kg. I Icm mh². Calculate the moment of inertia of a rectangular plate that is 240 cm long and 600 cm wide with a mass of 225 kg a.
When we take a situation when the axis passes through the centroid the moment of inertia of a rectangle is given as. Calculate the moment of inertia for a thin rectangular plate with the axis of rotation along the long edge. The moment of inertia of the rectangular plate is.
Viewed Along the Axis. Rectangular Plate about Axis Through the Edge or Thin Rod about Axis Perpendicular to Rod. Mass of the rectangular body density x volume.
Moments of Inertia for a slender rod with axis through center. Mass density of rectangle 15 gcm². The moment of inertia of a rectangle with respect to an axis passing through its centroid is given by the following expression.
Slender rod axis through center. For an axis along its shorter edge. Let its thickness be dy and s be the mass per unit volume of the plate.
I m a 2 3. I frac b h3 12 where b is the rectangle width and specifically its dimension parallel to the axis and h is the height more specifically the dimension perpendicular to the axis. Rectangular plate axis along edge.
Kinematics Moments of Inertia. I y 2 dM. Rectangular Plate Axis Along Edge.
The area of the rectangular plate is calculated as. Choose your coordinate system so that the axis of rotation blue line is along the y-axis and your area element dA b dx as 7. For an axis along its longer edge c.
Here b is used to denote the rectangle width the dimension parallel to the axis and h is said to be the height dimension perpendicular to the axis. Perpendicular Axis Theorem The moment of inertia MI of a plane area about an axis normal to the plane is equal to the sum of the moments of inertia about any two mutually perpendicular axes lying in the plane and passing through the given axis. Let the mass density be ρkgm 2Let the side PQRS a QRPS b From fig abMass of rectangle is m ρabTherefore Moment of Inertia of Rectangle about its center m 12a 2b 2 Distance of P point from center of rectangle is 2 a 2b 2 Therefore Moment of Inertia of Rectangle about P I m 12a 2b 2 m 4a 2b 2 m 3a 2b 2 Mass of triangle PQR 2m.
Show that its moment of inertia is 1-3 Ma where M is the mass of the plate and a is the length of the short length. M massh heightw widthMoment of Inertia of Thin Rectangular Plate I e. Find the value of x.
I m L 2 12. Moments of Inertia for a rectangular plane with axis along edge.

Find The Moment Of Inertia Of A Uniform Rectangular Plate Of Mass M And Edges Of Length I Youtube
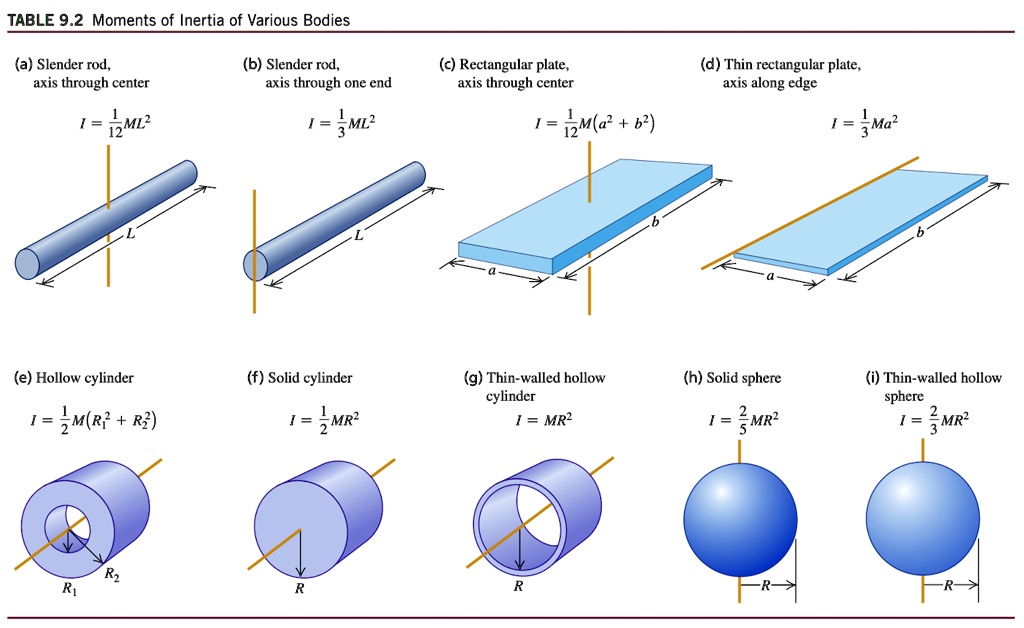
Solved Table 9 2 Moments Of Inertia F Various Bodies A Slender Rod Axis Through Center B Slender Rod Axis Through One End C Rectangular Plate Axis Through Center D Thin Rectangular Plate

0 Comments